internal energy, specifc heat capacity and specifc latent heat
Difficulty Levels:
1
Q&A
Medium
A heater supplies energy at a constant rate to $$0.045 \mathrm{~kg}$$ of a substance．The variation with time of the temperature of the substance is shown in Fig．3．1．The substance is perfectly insulated from its surroundings．
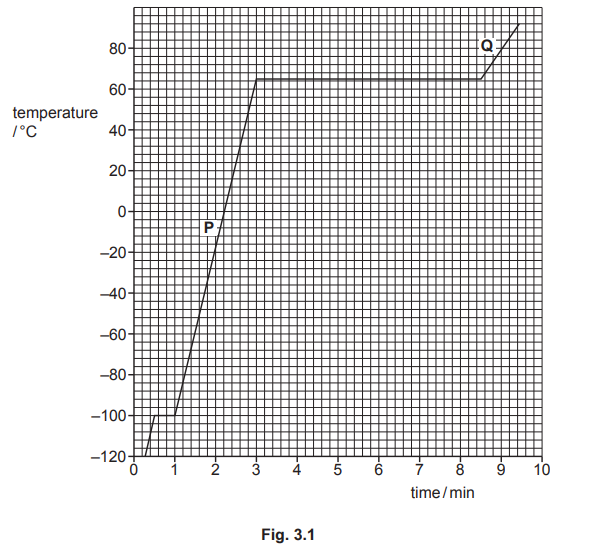（i）Determine the temperature at which the substance melts．
temperature $$=$$ $$\ldots \ldots \ldots \ldots \ldots \ldots \ldots \ldots { }^{\circ} \mathrm{C}$$
（ii）The power of the heater is $$150 \mathrm{~W}$$．
Use data from Fig．$$3.1$$ to calculate，in $$\mathrm{kJ} \mathrm{kg}^{-1}$$，the specific latent heat of vaporisation $$L$$ of the substance．
$$L=$$ $$\ldots \ldots \ldots \ldots \ldots \ldots \ldots \ldots $$ $$\mathrm{kJ} \mathrm{kg}^{-1}$$ [3]
（iii）Suggest what can be deduced from the fact that section $$\mathbf{Q}$$ on the graph is less steep than section $$\mathrm{P}$$
（i）Determine the temperature at which the substance melts．
temperature $$=$$ $$\ldots \ldots \ldots \ldots \ldots \ldots \ldots \ldots { }^{\circ} \mathrm{C}$$
（ii）The power of the heater is $$150 \mathrm{~W}$$．
Use data from Fig．$$3.1$$ to calculate，in $$\mathrm{kJ} \mathrm{kg}^{-1}$$，the specific latent heat of vaporisation $$L$$ of the substance．
$$L=$$ $$\ldots \ldots \ldots \ldots \ldots \ldots \ldots \ldots $$ $$\mathrm{kJ} \mathrm{kg}^{-1}$$ [3]
（iii）Suggest what can be deduced from the fact that section $$\mathbf{Q}$$ on the graph is less steep than section $$\mathrm{P}$$
Answer:
（i）$$-100^{\circ} \mathrm{C}$$
（ii）$$\begin{aligned} \text { time } &=8.5-3.0 \\ &=5.5 \mathrm{~min} \end{aligned}$$
$$P t=m L$$
energy $$=$$ power $$x$$ time $$=150 \times 5.5 \times 60$$
$$
=49500 \mathrm{~J}
$$
$$L=\frac{E}{m}$$
$$=\frac{49500}{0.045}$$
$$=1100 \mathrm{~kJ} \mathrm{~kg}^{-1}$$
（iii）gas has a higher specific heat capacity（than liquid）
Explanation:
2
Q&A
Medium
The specific latent heat of vaporisation is much greater than the specific latent heat of fusion for the same substance．
Explain this，in terms of the spacing of molecules．
Answer:
（much）greater increase in spacing of molecules（for vaporisation compared with fusion）
Explanation:
3
Q&A
Basic
Define specific heat capacity．[2]
Answer:
略
Explanation:
